SPECIAL CASE #2 A firstorder differential equation of the form y'=f (axbyc) where b0, can always be reduced to a separable firstorder equation by means of the substitution v=axbyc Example y'=1/ (xy1) Solution If we let v=xy1, then dv/dx=1dy/dx, so the differential equation is transformed into (dv/dx)1=1/v or dv/dx= (1v)/v, so2 solution(s) found x=1 y=0 See steps Step by Step Solution Rearrange Rearrange the equation by subtracting what is to the right of the equal sign from both sides of the equation (xy)*2(xy)*2(4*x*y)=0 Step by step solution Step 1Let R = ln(u2 v2 w2), u = x 2y, v = 2x − y, and w = 2xy Use the Chain Rule to find ∂R ∂x and ∂R ∂y when x = y = 1 Solution The Chain Rule gives ∂R ∂x = ∂R ∂u ∂u ∂x ∂R ∂v ∂v ∂x ∂R ∂w ∂w ∂x = 2u u2 v 2w ×1 2v u v 2w ×2 2w u v w2 ×(2y) When x = y = 1, we have u = 3, v = 1, and w = 2, so ∂R ∂x = 6 14 ×1 2 14 ×2 4 14 ×2 = 18
Ev3l9ptgm6uy7m
U-v=(x-y)(x^2+4xy+y^2)
U-v=(x-y)(x^2+4xy+y^2)-@v @x = @u @y @u @x = 3x 2 3y 4y;Simplifying 4xy(x y) = 0 (x * 4xy y * 4xy) = 0 Reorder the terms (4xy 2 4x 2 y) = 0 (4xy 2 4x 2 y) = 0 Solving 4xy 2 4x 2 y = 0 Solving for variable 'x' Factor out the Greatest Common Factor (GCF), '4xy' 4xy(y x) = 0 Ignore the factor 4 Subproblem 1 Set the factor 'xy' equal to zero and attempt to solve Simplifying xy = 0 Solving xy = 0 Move all terms containing x to the
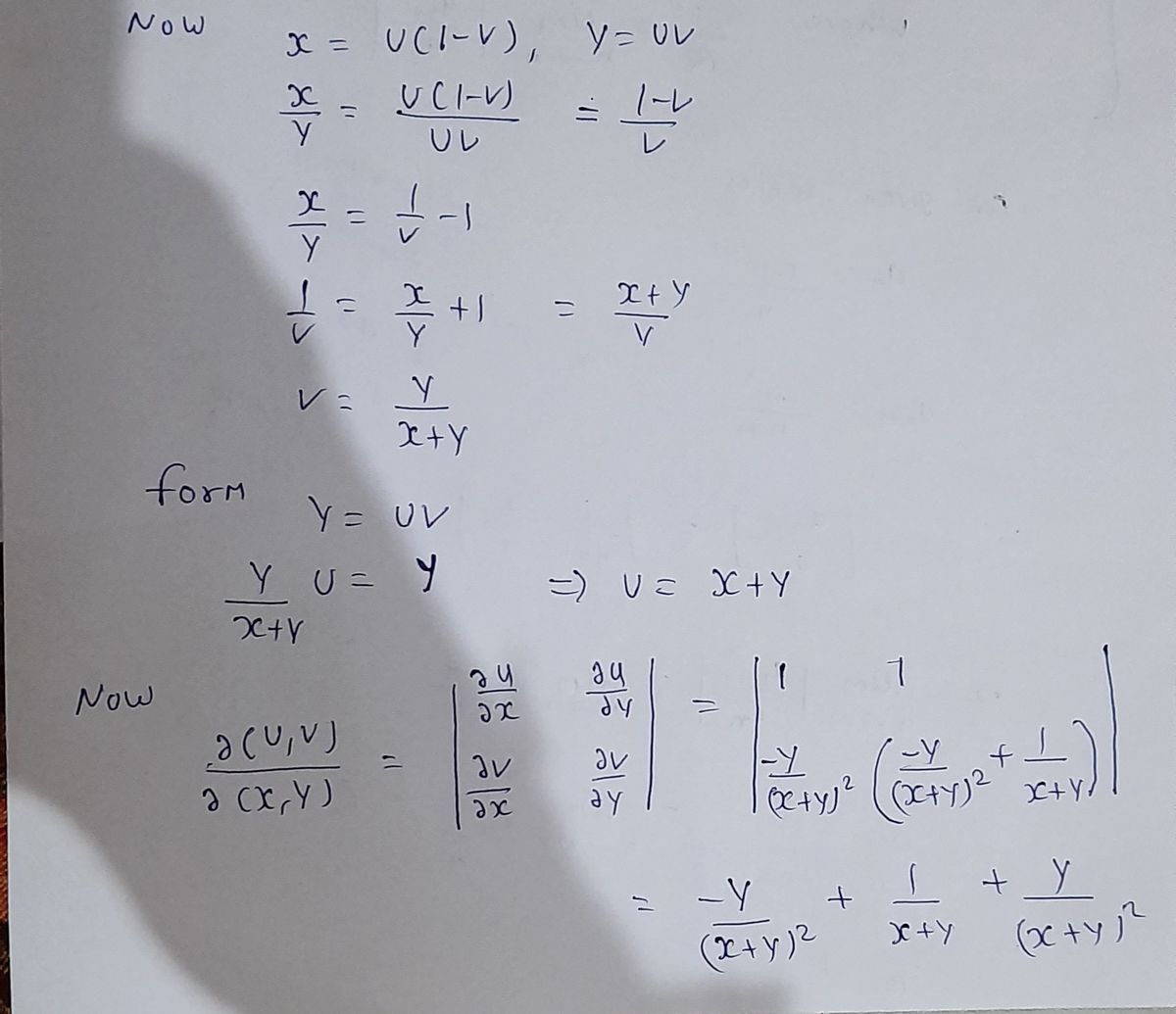



Answered If X U 1 V Y Uv Then Verify That Bartleby
They are "First Order" when there is only dy dx, not d 2 y dx 2 or d 3 y dx 3 etc Linear A first order differential equation is linear when it can be made to look like this dy dx P(x)y = Q(x) Where P(x) and Q(x) are functions of x To solve it there is a special method We invent two new functions of x, call them u and v, and say that y=uv We then solve to find u, and then find v, andU = x2 y2 2y ) ux = 2x = vy) v = 2xy ˚(x) ) vx = 2y ˚0(x) = uy = 2y 2 ) ˚(x) = 2xc ) f = x2 y2 2y i(2xy 2xc) where c is a real constant Let g = uiv We have v = 2xy y ) vy = 2x1 = ux) u = x2 x˚(y) ) uy = ˚0(y) = vx = 2y ) ˚(y) = y2 C ) g = x2 y2 xci(2xy y) where as before c is a real constant 3 Determine the domains where the following functions are holomorphic (i) f(z Excellent, Shovan Please note that substituting x by 2y in the expression asked we find the exact same answer, for sure
It is F(z) = u iv, F(z)cc = u iv u =( F Fcc )/2 , v =i ( F Fcc )/2 Follows u v =Re(1i)F(z) = Re(1i)(uiv) It is (1i)(uiv) = (uv) i( u vIn this math video lesson on Implicit Differentiation, I use implicit differentiation to find the derivative of 3x^2y^2=4x^24xy in terms of x and y #derivaMumbai University > First Year Engineering > sem 2 > Applied Maths 2 Marks 4 Year 14
y^2(x3)(x1) Given x^2y^24xy^23y^2 y^2(x^24x3) y^2(x^2x3x3) y^2(x(x1)3(x1)) y^2(x3)(x1) How do you find the two numbers by using the factoring method, if one number is seven more than Misc, 16 If (x iy)3 = u iv, then show that u/x v/y = 4 (𝑥2 – 𝑦2) We know that (𝑎 𝑏)^3 = 𝑎3 𝑏3 3𝑎𝑏 (𝑎 𝑏) Replacing a = x and b = iy (𝑥 𝑖𝑦)3= 𝑥3 (𝑖𝑦)3 3 𝑥 𝑖𝑦 (𝑥 𝑖𝑦) = 𝑥3 𝑖3𝑦3 3𝑥 𝑦𝑖 (𝑥 𝑖𝑦) = 𝑥3 𝑖2 ×𝑖 𝑦3 3𝑥2𝑦𝑖 3𝑥𝑦2𝑖2 Putting 𝑖2 = –1Simple and best practice solution for X^24xyy^2=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it Equation SOLVE Solution for X^24xyy^2=0 equation Simplifying X 2




Find The Minimum Value Of X 2 4xy 4y 2 2z 2 Given That Xyz 32 Mathematics Stack Exchange



Www Ualberta Ca Csproat Homework Math 215 Solution 7 Pdf
Given {eq}z = f\left ( x, y \right ), u = x^2 y^2 and v = 4xy {/eq} Find the value of {eq}\frac { \partial x}{\partial u} {/eq} {eq}\frac {Method 2 is for curves x(t), y(t) and surfaces x(u, v), y(u, v), z(u, v) with parameters A curve has one parameter t A surface has two parameters u and v (it is twodimensional) One advantage of parameters is that x, y, z get equal treatment, instead of picking out z as f(x, y) Here are the first two examples cone x = u cos v, y = u sin v, z = u cylinder x = cos v, y = sin v, z = u (4@u @y = 6xy 4x;




Pdf Partial Derivatives 13 1 Functions Of Several Variables Joseph Feliz Academia Edu



If X And Y Are Independent And Identically Distributed Uniform Random Variables On 0 1 Compute The Joint Density Of A U X Y V X Y B U X V X Y C U X Y V X X Y Homework Help
Must be solved to get u= g(x,y,c 2) on characteristics λ(x,y) = c 1, where c 2 is another constant of integration 3 The two arbitrary constants c 1 and c 2 can be thought of as being related by an arbitrary function c 2 = f c 1 12 Seven examples Example 1 Consider the simple PDE u x u y= 0 (112) Solution Obviously P= 1, Q= 1 and R= 0 Therefore the auxiliary equations (18) are dx 1Of course, (b) is the complete factorization, (a) is not Comparing the results in (a) and (b), we can get x 4 x 2 y 2 y 4 = (x 2 xy y 2)(x 2 –xy y 2) Further investigation x 4 x 2 y 2 y 4 = (x 4 2x 2 y 2 y 4) x 2 y 2 (note that one term is added and subtractedCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history



2
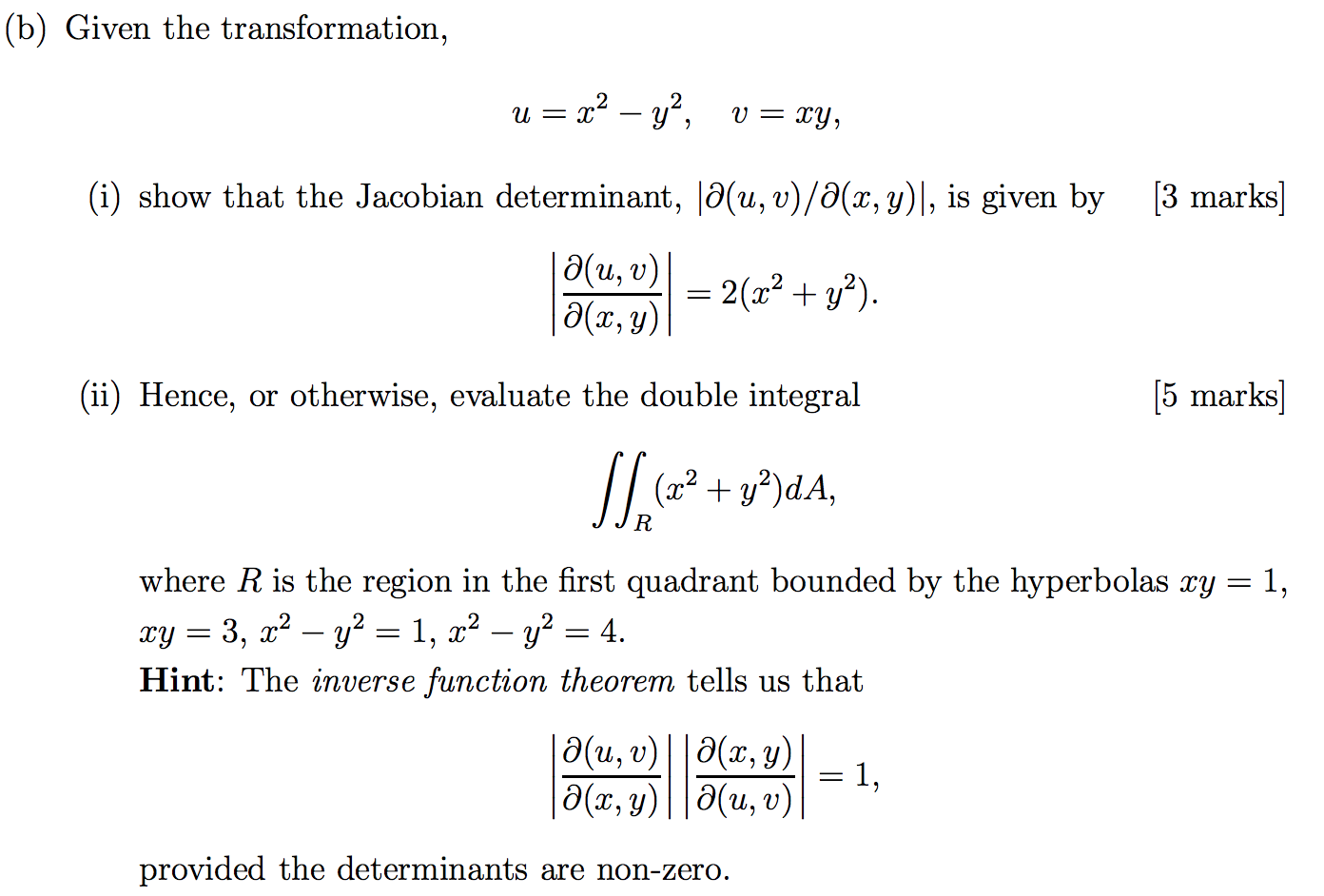



B Given The Transformation U X2 Y V My I Chegg Com
Substitute v = y x 1−2( y x)−( y x) 2 = 1 k 2 x 2 Multiply through by x 2 x 2 −2xy−y 2 = 1 k 2 We are nearly there it is nice to separate out y though!X24xy4y2 Final result (x 2y)2 Step by step solution Step 1 Equation at the end of step 1 ((x2) 4xy) 22y2 Step 2 Trying to factor a multi variable polynomial 21 Proof that x^24xyy^2=1 has infinitely many integer solutionsThe given equation is x² 4xy y² = On differentiating w r t x we get, d/dx( x² 4xy y² ) = d/dx() 2x (4x)(dy/dx) 4y (2y)(dy/dx) = 0 On apply product rule (




J Osxal Osx Y 1 Q F X Y 24xy 1 Find Pc Y A Find Joint Density 111 Homeworklib



Differential Calculus Ii New Age International
Problem 2 Determine the global max and min of the function f(x;y) = x2 2x2y2 2y2xy over the compact region 1 x 1;(1228) y e x 2cos 2x 1 2 sin 2x Case of a double root If the discriminant a2 4b 0, then the auxiliary equation has one root r, which gives us only one solution erx of the differential equation We find another solution by the technique of variation of parameters We try y uerx, where u is a new unknown function Now, the differential equation is (1229) y 2ry r 2y 0 Substituting this y in22 4x 2 4xy y 2 is a perfect square It factors into (2xy)•(2xy) which is another way of writing (2xy) 2 How to recognize a perfect square trinomial • It has three terms • Two of its terms are perfect squares themselves • The remaining term is twice the product of the square roots of the other two terms Final result (2x y) 2
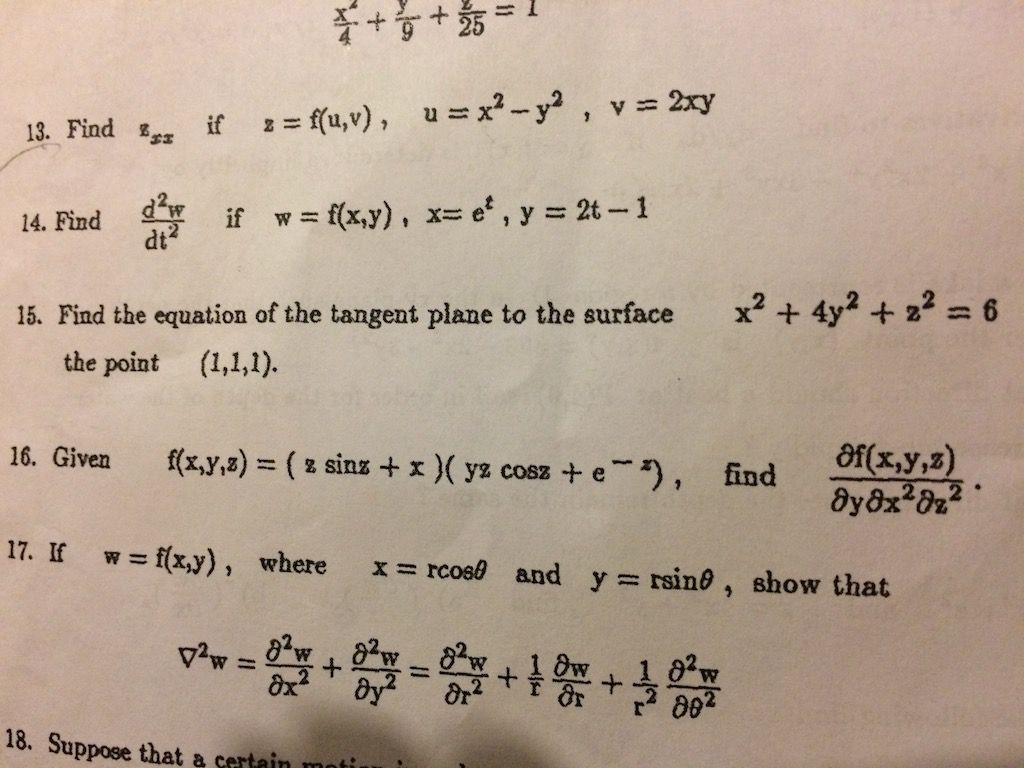



13 Find Zss If Z T U V U X2 Y2 V 2xy 14 Find Chegg Com




Cauchy Riemann Show That U V X Y X2 4xy Y2 Find F Z Youtube
uv=(xy)(x^24xyy^2)2(xy),求f(z)=uvi 展开 我来答 1个回答 #热议# 东京奥运会,能给日本带来什么好处? 杨雪灵乖乖 TA获得超过335个赞 知道小有建树答主 回答量: 316 采纳率: 100% 帮助的人: 436万 我也去答题 访问个人页 展开全部 学霸君 更多追问追答 追答 猿题库 小猿搜题 已赞The velocity of a flow field is defined by u= (2x^2−y^2)m/s and v= (−4xy)m/s, where x and y are in meters Determine the magnitude of the velocity and acceleration of a particle that passes through point 11 m, 1 m2 Find the equation of the streamline passing through thisIn calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivativeIt is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more easily found




Math Complex Analysis Holomorphic Function



Ocw Mit Edu Resources Res 18 007 Calculus Revisited Multivariable Calculus Fall 11 Study Materials Mitres 18 007 Partiii Sol06 Pdf
设u及v是解析函数f (z)的实部及虚部,且uv= (xy) (x^24xyy^2)z=xiy,求f (z)We can try to factor x 2 −2xy−y 2 but we must do some rearranging first Change signs y 2 2xy−x 2 = − 1 k 2 Replace − 1 k 2 by c y 2 2xy−x 2 = c Add 2x 2 to both sides y 2 2xyx 2 = 2x 2 c Factor (yx) 2Question (6' ) Suppose That U(x ,y ) v (x ,y ) =(xy)(x24xyy2)2(xy) Determine The Analytic Function F(z)=u(x,y) Iv(x,y) This problem has been solved!



Math 251 Diary Fall 10




3 Inner Product Spaces Pdf Free Download
U(x;y) = x3 3xy2 4xy Show that uis harmonic and determine the harmonic conjugate v(x;y) satisfying v(0;0) = 0 For this function uand for the harmonic conjugate vjust determined express the function f(z) = u(x;y) iv(x;y) in terms of zonly Solution The Cauchy Riemann equations are @u @x = @v @y;(x−y)2 Example 6 U =(x−3y)3 Answer ∂U ∂x = U x =3(x−3y)2(1) = 3(x−3y)2 ∂U ∂y = U y =3(x−3y)2(−3) = −9(x−3y)2 3 A Special Function CobbDouglas The Cobbdouglas function is a mathematical function that is very popular in economic models The general form is z = xayb and its partial derivatives are ∂z/∂x = axa−1yb and ∂z/∂y = bxayb−1 Furthermore, the slopeIf x2 4y2 = 4xy, then x y is a) 1 1 b) 1 2 c) 2 1 d) 1 4




18 G12 Math E Flip Book Pages 351 400 Pubhtml5




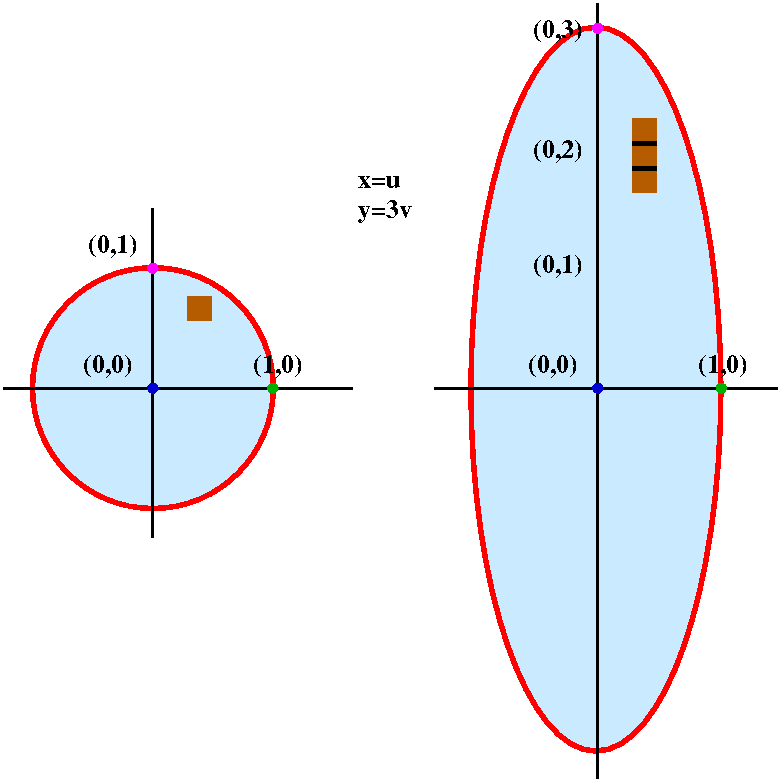
Answered 9 Let R Be The Region In The First Bartleby
X 2 y 2 10x 2y 4xy = 10 Start by differentiating both sides by x, the terms not containing y are differentiated normally, x 2 becomes 2x, 10x becomes 10, and 10 becomes 0 For the y 2 term, by implicit differentiation the result is 2y (the same as with x) multiplied by a factor of dy/dx, or 2y(dy/dx) For the 2y term, as above, the result is the same as for x, 2 in this caseSolve for x Use the distributive property to multiply xy by x^ {2}xyy^ {2} and combine like terms Use the distributive property to multiply x y by x 2 − x y y 2 and combine like terms Subtract x^ {3} from both sides Subtract x 3 from both sides Combine x^ {3} and x^ {3} to get 0 Combine x 3 and − x 3 to get 0 ux = 3x2 2y − 4y2 uy = 2x − 8xy after applying CauchyRiemann Equations vy = ux = 3x2 2y − 4y2 and after integration v(x, y) = 3x2y y2 − (4 / 3)y3 β(X) and after trying to solve for β I found it equal to β = x2y − x2 and after applying it to the
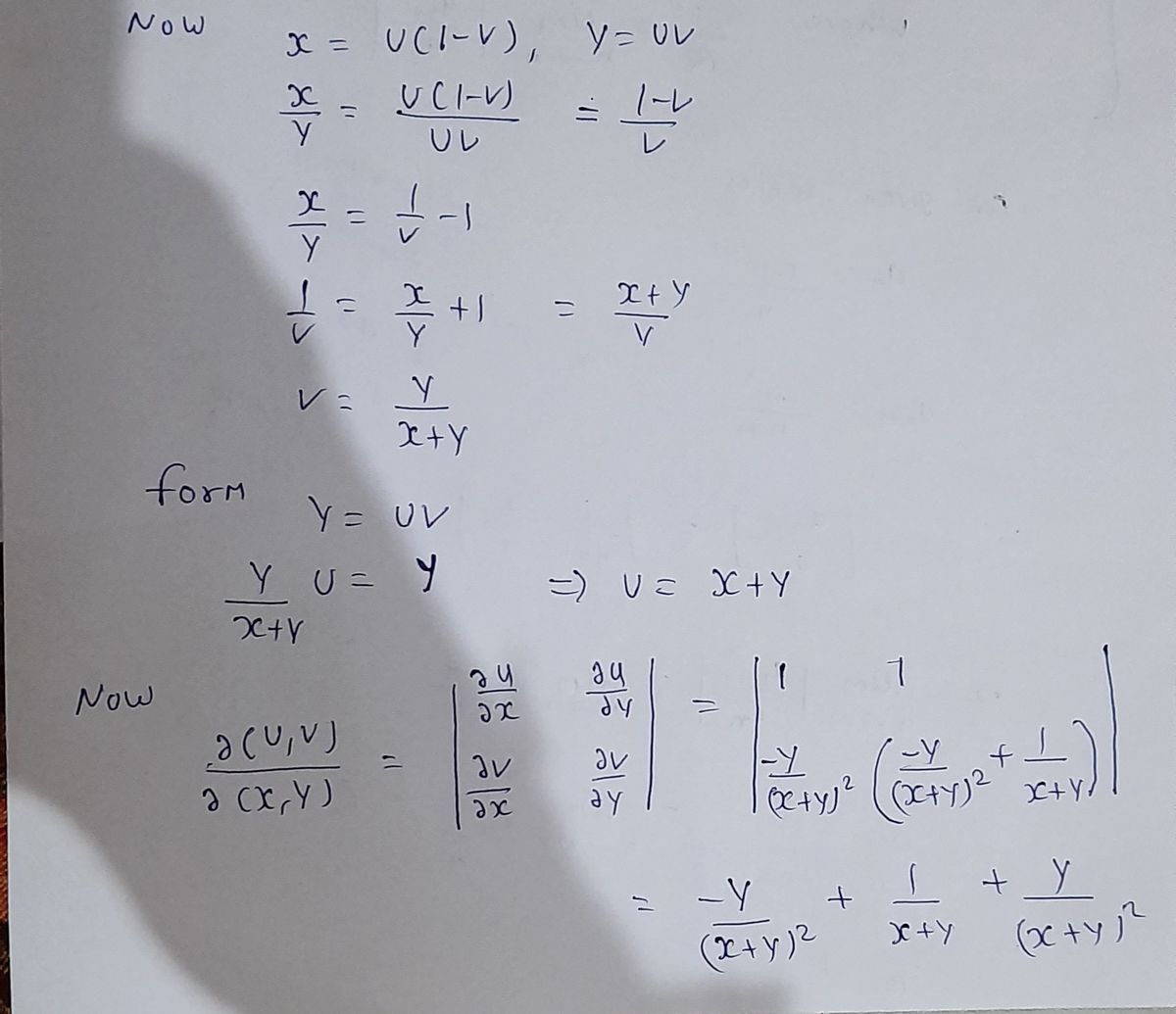



Answered If X U 1 V Y Uv Then Verify That Bartleby
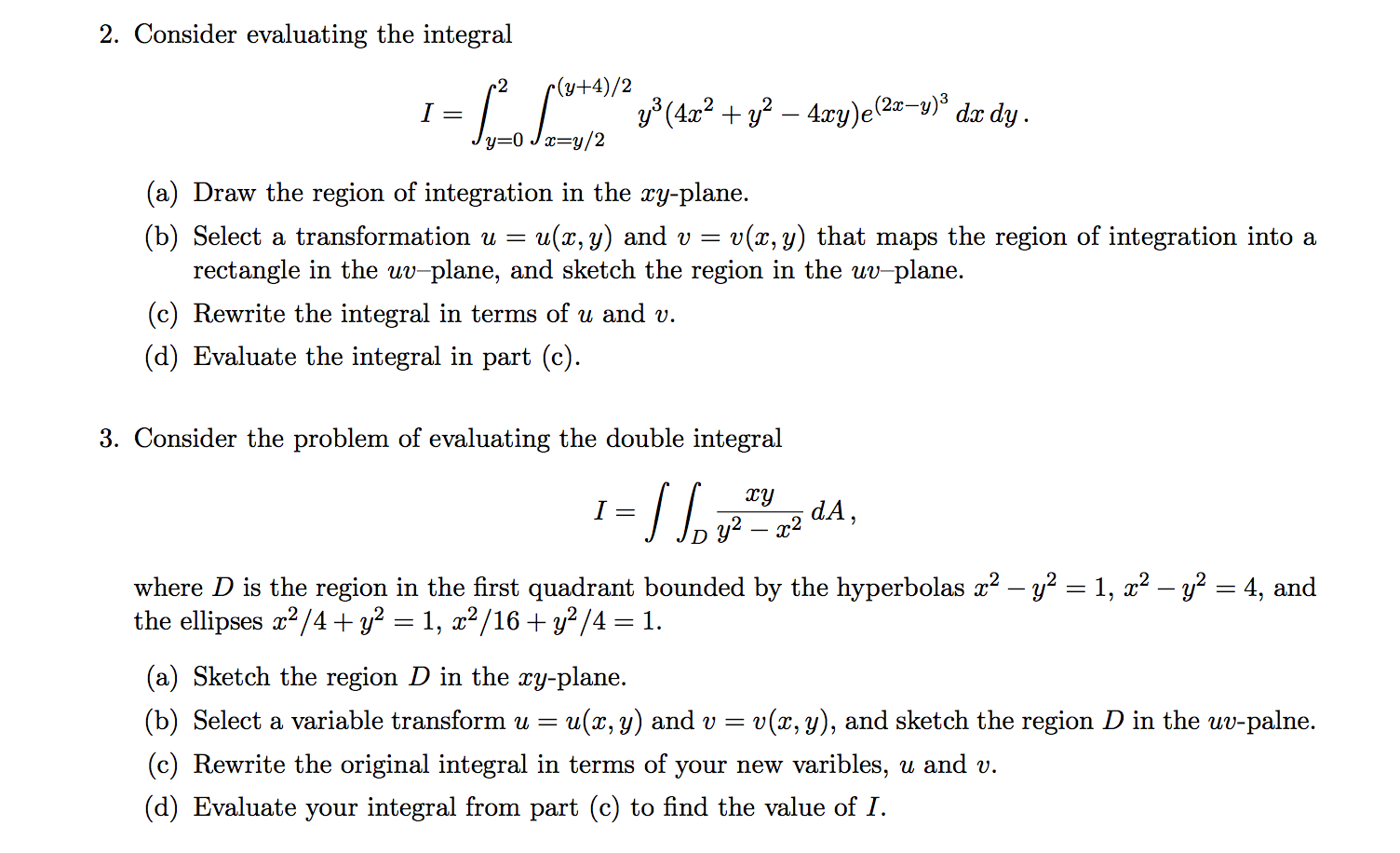



Consider Evaluating The Integral I Integral 2 Y 0 Chegg Com
A horizontal velocity field is defined by u = 2(x^2 y^2) ft/s and v = (4xy) ft/s Show that these expressions satisfy the continuity equation Using the Navier–Stokes equations, show that the pressure distribution is defined by p = C \rho V^2/2 \rho gz19,877 results, page 17 ELA Analyzing Literary Text Write an Essay The story The Swallow and the Pumpkinseed is a folktale and Don't Count Your Chickens is written in the style of a folktale In many0 y 2 Solution We look for the critical points in the interior



Http Www Math Ntu Edu Tw Cheng Teaching Calculus Ch15 Pdf




If X And Y Are Independent And Identically Distributed Uniform Random Variables On 0 1 Compute The Joint Density Of A U X Y V X Y B U X V X Y C U X Y V X X Y Homework Help
= (x y)(x 2 – xy y 2)(x y)(x 2 xy y 2), by (2) and (3) Which of the above factorization is correct?Topics Terms and topics; (1) u(x,y)=x^2y^2 ∂u/∂x=2x ∂u/∂y=2y 正則であるための必要条件、コーシーリーマンの関係式から ∂v/∂y=2x より v(x,y)=2xyf(x) ∂v/∂x=2y より v(x,y)=2xyg(y) 上の2式より v(x,y)=2xyC このとき、u(x,y)とv(x,y)が全微分可能は明らかだから、求める正則関数は f(z)=(x^2y^2)(2xyC)√(1) (2) u(x,y)=(xy)(x^24xyy^2) ∂u/∂x



2




Double Integration
Find dy/dx x^24xyy^2=4 Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps Differentiate Tap for more steps By the Sum Rule, the derivative of with respect to is Differentiate using the Power Rule which states that is where Evaluate Tap for more steps Since is constant with respect to , the derivative of with respect to isSeparabledifferentialequationcalculator y'4xy^{2}=x en Related Symbolab blog posts Advanced Math Solutions – Ordinary Differential Equations Calculator, Bernoulli ODE Last post, we learned about separable differential equations In this post, we will learn about BernoulliTranscribed image text 2 (a) Find an analytic function f(x) = u(x, y) iv(x, y), where u v= = (x – y)(x2 4xy y2) 12M (b) Let f(z) = u(x, y) iv(x, y) be an anlaytic function defined on C Then show that a2 O) 15(2)/2 = 415%(2)12 Dx2 for all z EC 10M




Cauchy Riemann Show That U V X Y X2 4xy Y2 Find F Z Youtube



2
If u = x 2 − y 2, v = 2 x y a n d z = f ( u, v) prove the following written 50 years ago by shailymishra30 ♦ 330 modified 14 months ago by sanketshingote ♦ 570 ( ∂ z ∂ x) 2 ( ∂ z ∂ y) 2 = 4 u 2 v 2 ( ∂ z ∂ u) 2 ( ∂ z ∂ v) 2 partial differentiation ADD COMMENTStack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange uv=(xy)(x^24xyy^2)2(xy),求f(z)=uvi 展开 我来答 1个回答 #专题# 日本人为什么抵制东京奥运会? shit7 超过17用户采纳过TA的回答 知道答主 回答量: 68 采纳率: 0% 帮助的人: 1万 我也去答题 访问个人页 关注 展开全部 几年级的题 已赞过 已踩过 你对这个回答的评价是? 评论




Find The Limit X Y Rightarrow 1 1 2x 2 Chegg Com
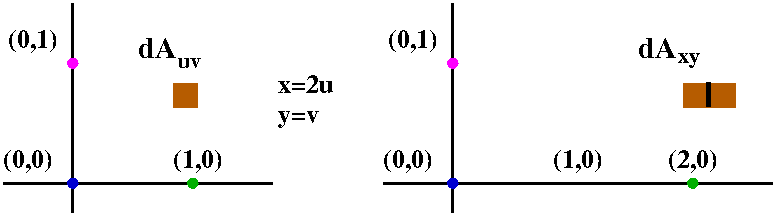



Math 251 Diary Fall 10
Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorY(x2 xy 3)dydx Example 2 X and Y are jointly continuous with joint pdf f(x,y) = ˆ cxy if 0 ≤ x, 0 ≤ y, xy ≤ 1 0, otherwise (a) Find c (b) Find P(Y > X) (c) Find marginal pdf's of X and of Y (d) Are X and Y independent (justify!) We start (as always!) by drawing the support set (See below, left) y y 1 y=x 1 x 1 x 1 y=1−x of support set with y>x Blue subset support set4xy′′ 2(−2√x 1)y′ y = 0, with initial values y(0) = 1, y(1) = 2e (Hints make change of variable y = v, x = u2, convert it to an constant coefficient ODE ) What is y?




Millersville University Name Answer Key Department Of Mathematics




Iii Ec Eee Ice Bm Ii Assignment Trigonometric Functions Mathematical Analysis
(xy)2(xy)2=4xy This deals with linear equations with one unknown Overview;



Cauchy Riemann Show That U V X Y X2 4xy Y2 Find F Z Youtube



Http Edshare Soton Ac Uk Id Document 6767




Section 15 4 Answers Derivative Tangent



Www3 Nd Edu Zxu2 Triple Int16 8 Pdf



2



14 7 Change Of Variables In Multiple Integrals Jacobians Mathematics Libretexts



Ocw Mit Edu Resources Res 18 007 Calculus Revisited Multivariable Calculus Fall 11 Study Materials Mitres 18 007 Partiii Sol06 Pdf



Www Rgpvonline Com Guide Notes M3 Unit 1 Pdf




If X2 Y2 2 Xy Find Dy Dx Mention Each And Every Step Mathematics Topperlearning Com Gwvwfskk
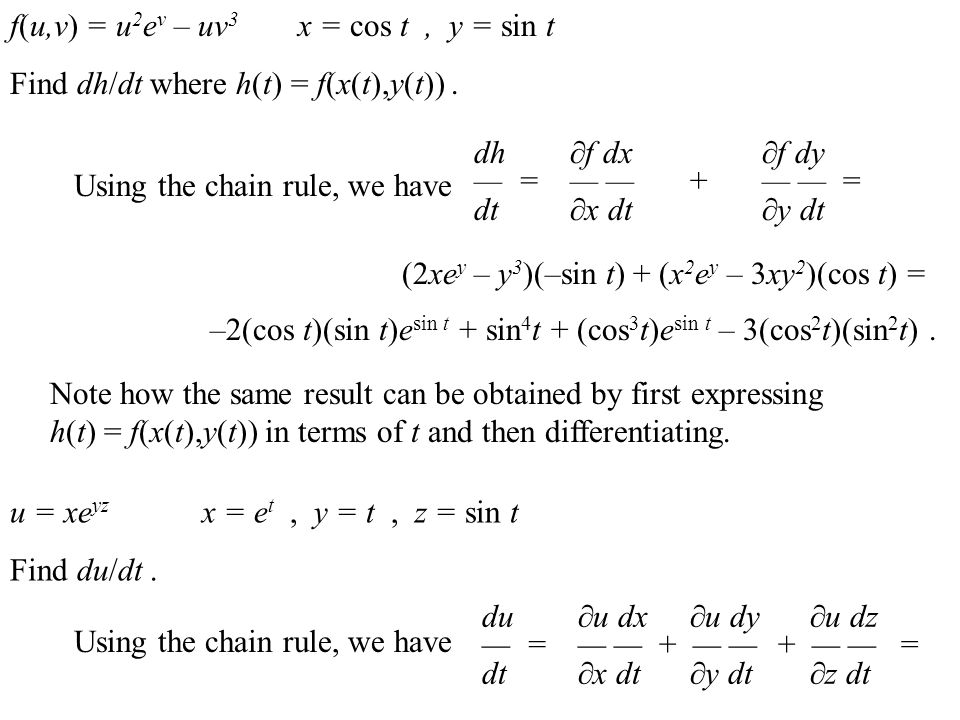



The Derivative Rules For Multivariable Functions Stated Theorem 10 On Page 151 Are Analogous To Derivative Rules From Single Variable Calculus Example Ppt Download




Let R Be The Region In The First Quadrant Of The Chegg Com
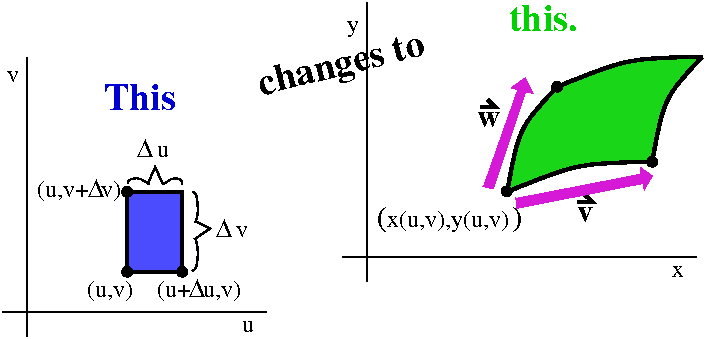



Math 251 Diary Fall 10



Ev3l9ptgm6uy7m




Tinkutara Equation Editor Math Forum Question




Jacobian Find ꝺ U V ꝺ R 8 If U 2xy V X 2 Y 2 Where X Rcos8 Y Rsin8 Youtube




21 Find The Jacobian Of The Transformation From The Chegg Com



What Is The General Solution Of The Ydx Xy 2 X Y Dy 0 Quora



Ocw Mit Edu Resources Res 18 007 Calculus Revisited Multivariable Calculus Fall 11 Study Materials Mitres 18 007 Partiii Sol06 Pdf



How To Solve For X And Y In Terms Of U And V When U Xy And V Xy 3 Quora




50 Zƒ ƒvƒ Xy Z Z V W Xy




2 Functional Dependence Differential Calculus Functions And Mappings
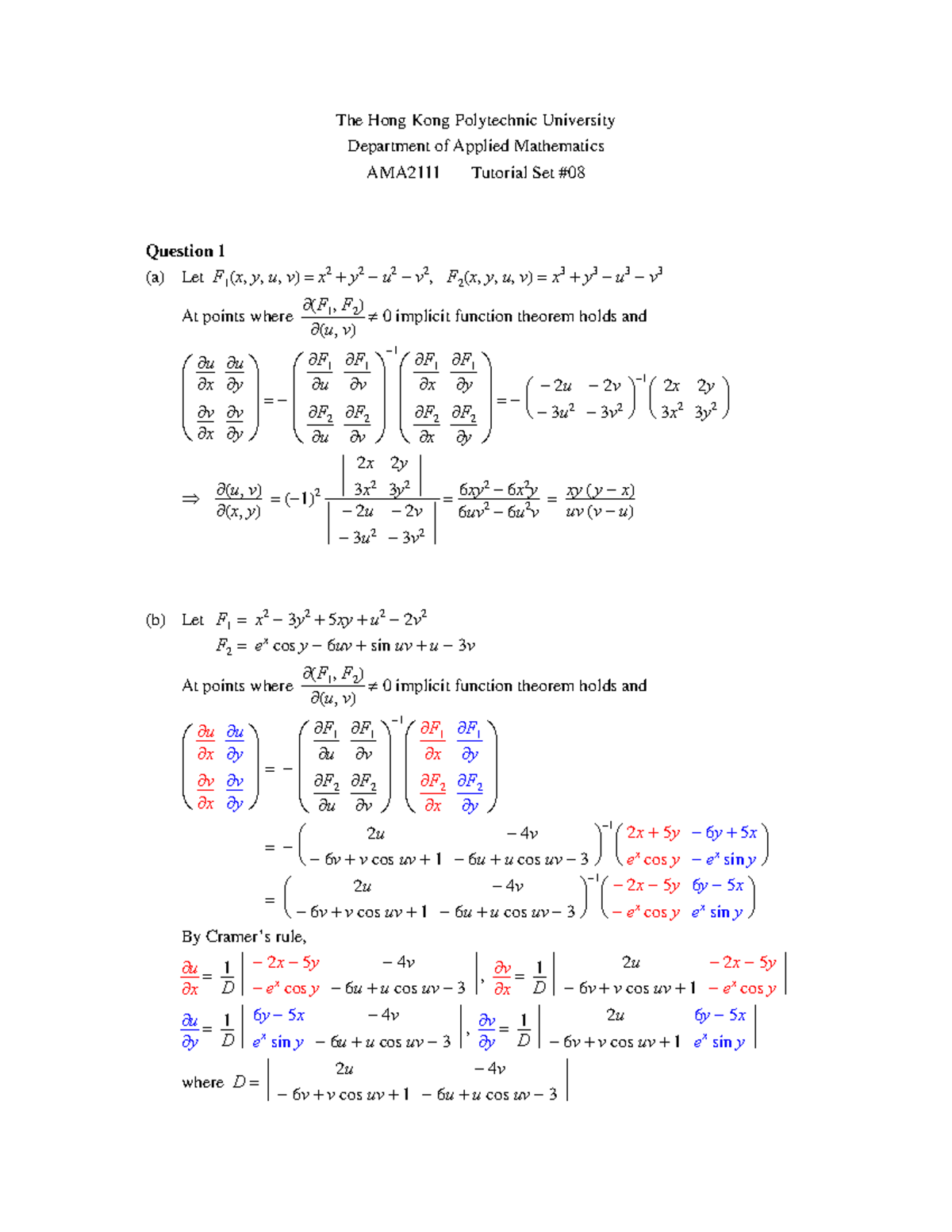



Sol8 Ama2111 Notes Studocu




Calc 501 1000 By James Bardo Issuu




2 Functional Dependence Differential Calculus Functions And Mappings
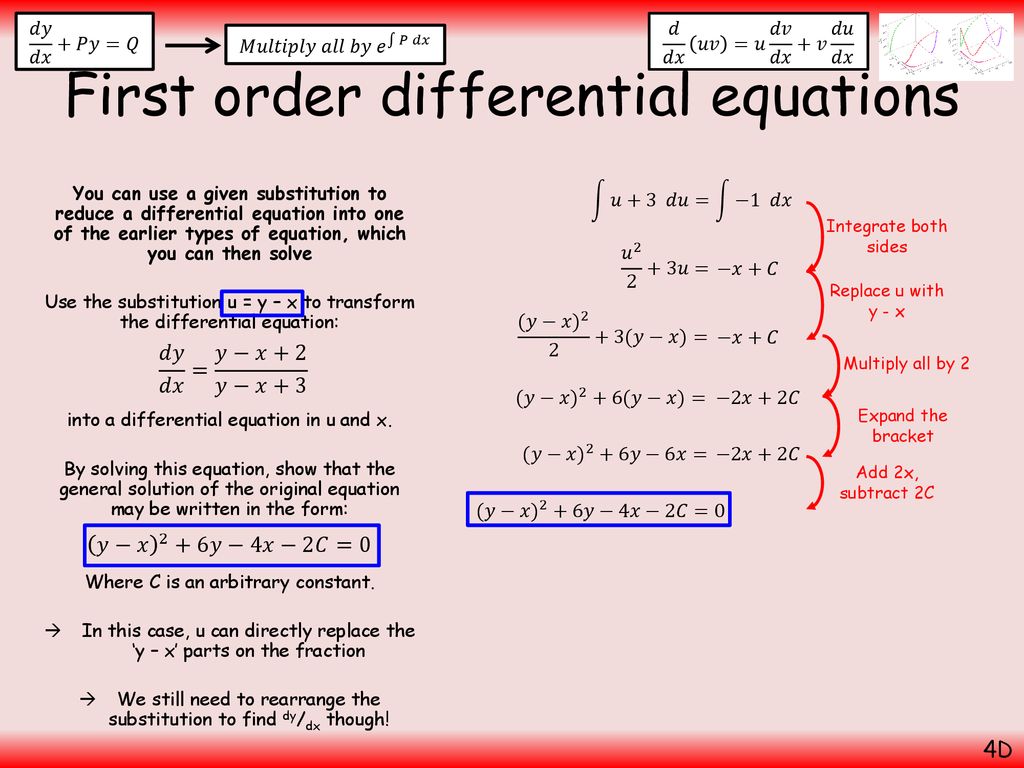



Differential Equations Ppt Download




Jacobians New



Http Web Yonsei Ac Kr Ezaid Board Download Aspx Method Download Pfkhomepageno 1710 Fkboardentrypkno 8 Attachefilechoice 1 Pkno




Can U Plzz Send The Solution Of These Problems Please Chegg Com



Www Rgpvonline Com Guide Notes M3 Unit 1 Pdf
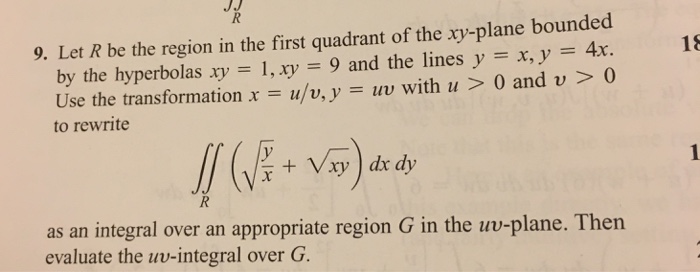



Let R Be The Region In The First Quadrant Of The Chegg Com




J Xy



2




1 Consider Two Random Variables X And Y With Joint Density Function F X Y 12xy 1 Y 0 X 1 0 P 1 Otherwise Homeworklib




2 Partial Differentiation



2




How Do You Implicitly Differentiate 4 2x Y 2 Y 2x 3x 2y Socratic




Divergence Theorem Of Gauss Example 1 Example 2



1365 Exam Studocu



2



Http Www Math Sci Hokudai Ac Jp S Settepanella Teachingfile Calculus Calculus2 Pagine Lineintex Pdf
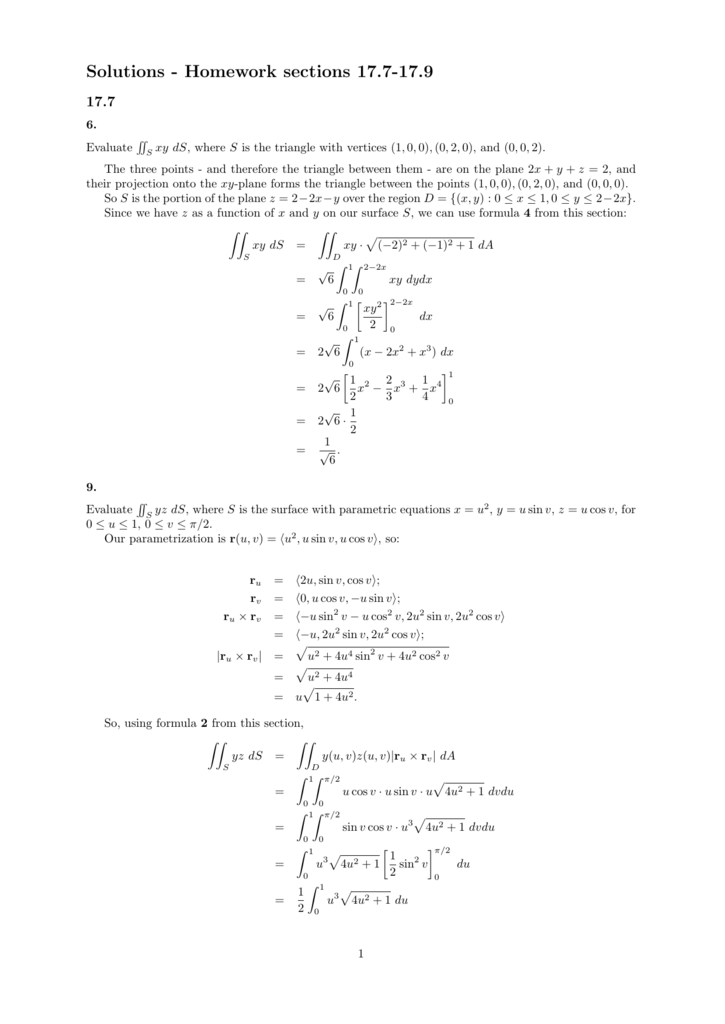



Solutions Homework Sections 17 7 17 9
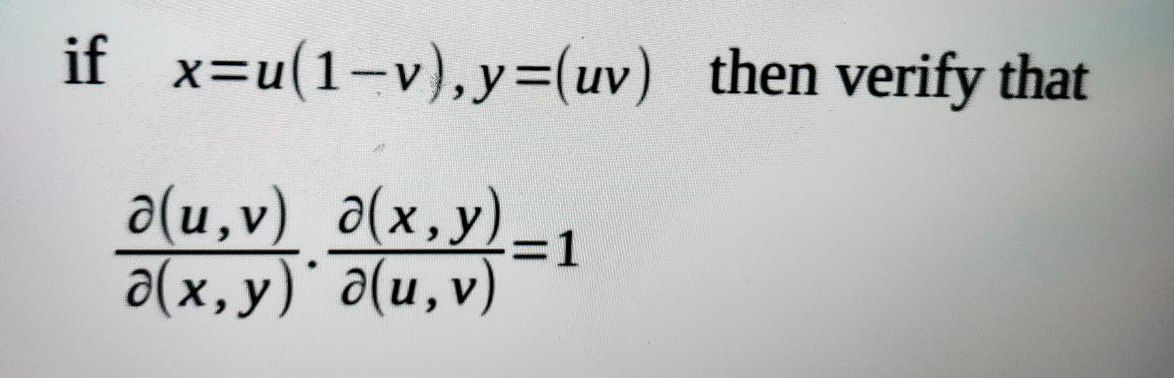



Answered If X U 1 V Y Uv Then Verify That Bartleby




Seminar Week 1 Solutions Studocu



Solved In The Xy Plane Let R Be The Square With Vertices 0 0 1 1 2 0 1 1 A Find Equations For A Transformation T That Maps A Re Course Hero



2




Apply The Transformation U 2x Y V 3 2 2y To Chegg Com




2 Functional Dependence Differential Calculus Functions And Mappings



Web Maths Unsw Edu Au Cct Math19 Problems Answers Pdf



2




Cauchy Riemann Show That U V X Y X2 4xy Y2 Find F Z Youtube



2
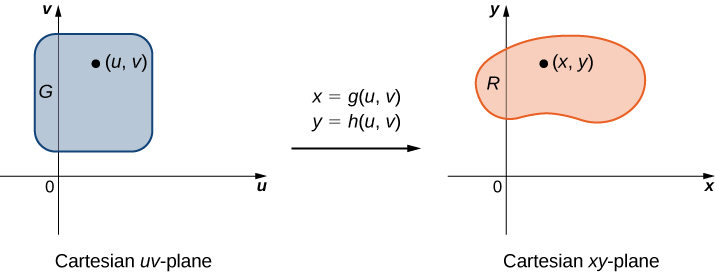



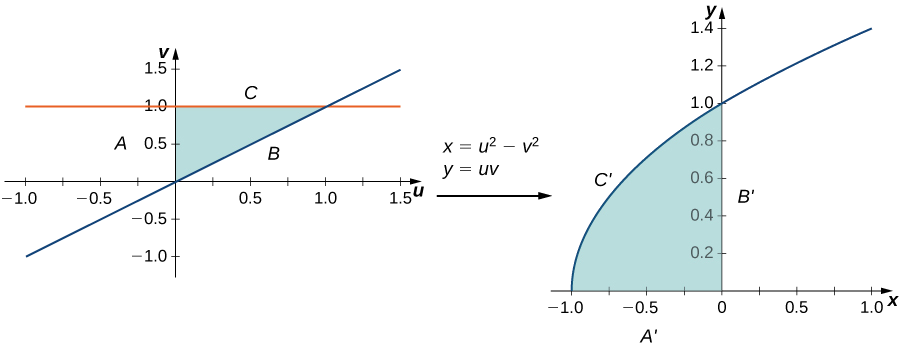
Change Of Variables In Multiple Integrals Calculus Volume 3




18 G12 Math E Flip Book Pages 351 400 Pubhtml5




Cauchy Riemann Show That U V X Y X2 4xy Y2 Find F Z Youtube




Pdf Ee6 Solutions Assignment 1 Mauricio Miguel Matute Estrada Academia Edu
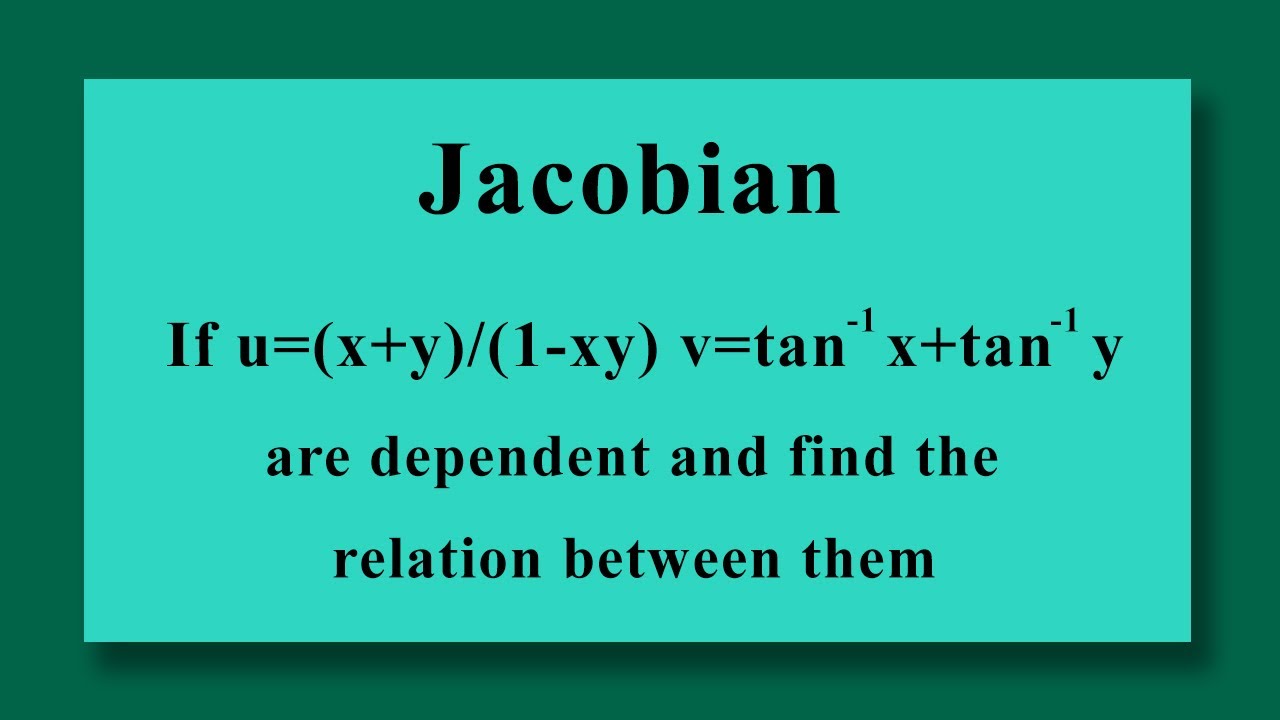



Jacobian Show Function U X Y 1 Xy V Tan 1 X Tan 1 Y Functionally Dependent Find Relation Youtube



Www Rgpvonline Com Guide Notes M3 Unit 1 Pdf



Multiple Integrals H 2 Y Are Continuous Functions On C D And Let F X Y Be A Function Defined On R Then Pdf Free Download



If U V X Y X2 4xy Y2 And F Z U Iv Is An Analytic Function Of Z X Iy Find F Z Is Terms Of Z Brainly In



Http Www Math Sci Hokudai Ac Jp S Settepanella Teachingfile Calculus Calculus2 Pagine Lineintex Pdf



Www Math Tamu Edu Kahlig Notes 251 Ch15 9 Pdf




Solutions Homework Sections Pdf Free Download




4 1 5x 8x3x 2 7y9y 2y 3 1 See How To Solve It At Qanda
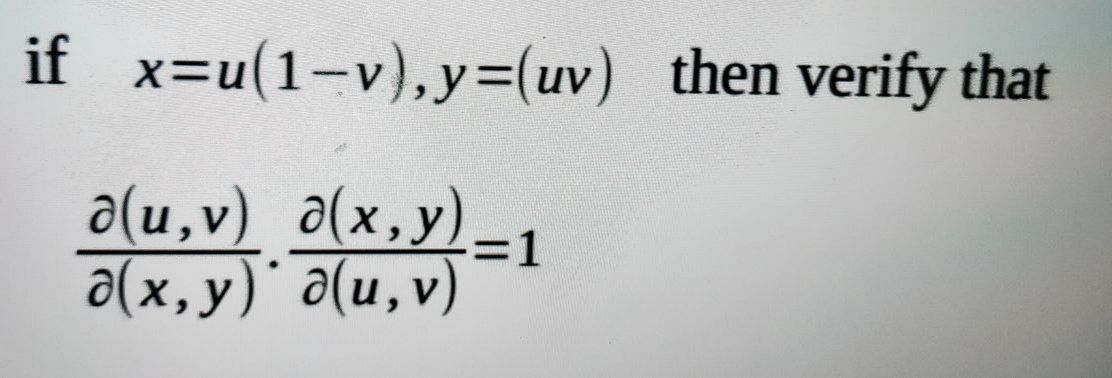



Answered If X U 1 V Y Uv Then Verify That Bartleby



Http Www Math Drexel Edu Rboyer Courses Math0 Summer 14 Final Review Problems Pdf




Please Solve These Qns Maths Meritnation Com




The Region R Is Bounded By The Following Curves X2 Y2 4 X2 Homeworklib



Math Dartmouth Edu M13s17 M13s17 Hw4 Sol Pdf



File



Http Math Oit Edu Debd Teaching W Math354 Practice Set For Final Exam Pdf



2




Ab2 5 Surfaces And Surface Integrals Divergence Theorem Of Gauss Pdf Free Download



0 件のコメント:
コメントを投稿